Shanghai Institute of Optics and Fine Mechanics----Coherent Ghost Imaging Based on Sparsity Constraint Without Phase-sensitive Detection

Coherent Ghost Imaging Based on Sparsity Constraint Without Phase-sensitive Detection

Update time： 2012-05-24

Ghost Imaging (GI) is a novel and promising technique that can reconstruct the object’s real space image or its Fourier-transform diffraction spectrum based on the classical or quantum correlation of the light field fluctuations. GI has benefited lots of practical applications with its particular scheme advantages. However, the traditional GI process of calculating the correlation is little efficient, and massive measurements are required for good visibility.

Recently, efforts have been made to combine GI with sparsity reconstruction. This combination makes great sense because it preserves not only the scheme advantage of GI but also higher efficiency and even super-resolution.　

Researchers at Key Laboratory for Quantum Optics and Center for Cold Atom Physics of CAS, Shanghai Institute of Optics and Fine Mechanics, Chinese Academy of Sciences (SIOM, CAS) recently presents a universal process for coherent GI without phase-sensitive detection. The process is based on the sparsity constraint of the target, which helps to accelerate the information extraction. By taking advantage of this process, the coherent GI scheme with a point-like detector in the test path is improved to achieve higher efficiency and higher resolution, even though the phase information of the random field is lost. Experimental results of diffraction GI via such process are presented to show higher efficiency and higher resolution compared with traditional GI results. This process will contribute to the practical applications, such as Fourier-transform diffraction GI of X-ray, and remote sensing.

This work entitled " Coherent Ghost Imaging based on sparsity constraint without phase-sensitive detection " has been published in Europhysics Letters, 98 (2012) 24003.(http://iopscience.iop.org/0295-5075/98/2/24003/)

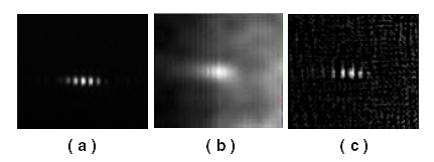Fig.1 The experimental spectrum reconstructions of a double slit. (a) spectrum realized by a lens in a f-f system; (b) spectrum by traditional correlated GI with 1000 measurements; (c) spectrum by sparsity reconstruction with the same measurements as in (b).(Imagy by SIOM)

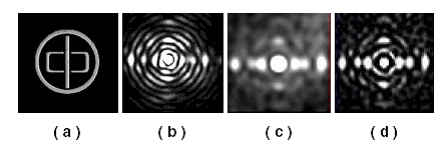Fig.2 The experimental spectrum reconstructions of a “Zhong” ring. (a) The object; (b) spectrum realized by a lens in a f-f system; (c) spectrum by traditional GI with 4000 measurements; (d) spectrum by sparsity reconstruction, with the same measurements as in (c).(Imagy by SIOM)

附件下载：